¡Tu solución está lista!
Nuestra ayuda de expertos desglosó tu problema en una solución confiable y fácil de entender.
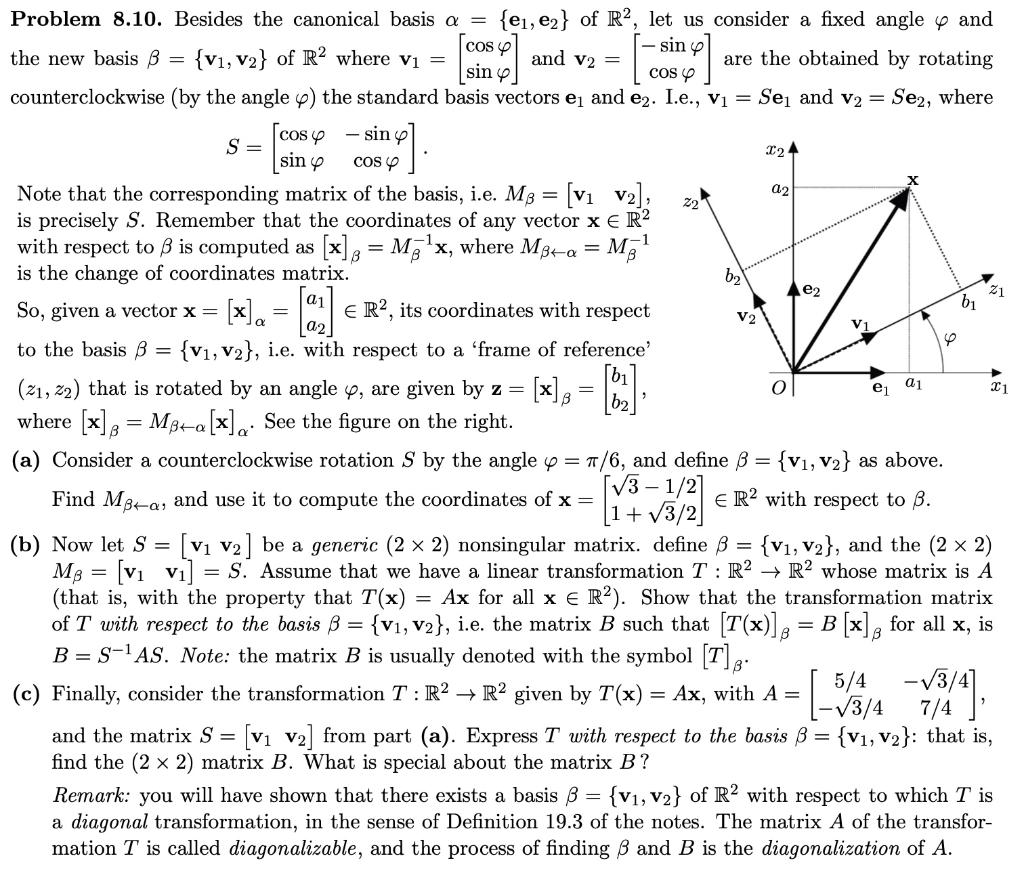
Mira la respuestaMira la respuesta done loadingPregunta: [ sin y [cos y sin 12 a2 = X 21 b1 = V2 Vi = = [a)
- Esta es la mejor manera de resolver el problema.Solución
Texto de la transcripción de la imagen:
[ sin y [cos y sin 12 a2 = X 21 b1 = V2 Vi = = [a) <R?, its coordinates with respect - Level = 0 ei a 1 21 Problem 8.10. Besides the canonical basis a = {el, ez} of R2, let us consider a fixed angle y and cos - sin the new basis B = {V1, V2} of R2 where v1 = and V2 = are the obtained by rotating COS counterclockwise (by the angle ) the standard basis vectors e, and e2. I.e., V1 = Sej and v2 = Se2, where - sino S= COS Note that the corresponding matrix of the basis, i.e. MB = [v1 v2], is precisely S. Remember that the coordinates of any vector x € R2 with respect to B is computed as [x]2 = Mā'x, where Møta = M 1 is the change of coordinates matrix. b2 So, given a vector x= [x]. E to the basis B {V1, V2}, i.e. with respect to a 'frame of reference' (21, 22) that is rotated by an angle y, are given by z = [x] where [x] 2 = MBa[x]c. See the figure on the right. (a) Consider a counterclockwise rotation S by the angle y = 7/6, and define B = {V1, V2} as above. [V3 - 1/2] Find Mera, and use it to compute the coordinates of x= ER2 with respect to B. [1 + V3/2] (b) Now let S = [ ví v2] be a generic (2 x 2) nonsingular matrix. define B = {V1, v2}, and the (2 x 2) V1 MB = (v1 vi] = S. Assume that we have a linear transformation T:R2 + R2 whose matrix is A (that is, with the property that T(x) = Ax for all x € R2). Show that the transformation matrix of T with respect to the basis B = {V1, V2}, i.e. the matrix B such that (T(x)]g = B [x]g for all x, is B = S-AS. Note: the matrix B is usually denoted with the symbol (T]2. 5/4 -V3/ (c) Finally, consider the transformation T: R2 + R2 given by T(x) = Ax, with A = -V3/4 and the matrix S= [v1 v2] from part (a). Express T with respect to the basis B = {V1, V2}: that is, find the (2 x 2) matrix B. What is special about the matrix B? Remark: you will have shown that there exists a basis B = {V1, V2} of R2 with respect to which T is a diagonal transformation, in the sense of Definition 19.3 of the notes. The matrix A of the transfor- mation T is called diagonalizable, and the process of finding B and B is the diagonalization of A. = B 10 = B B
Estudia mejor, ¡ahora en español!
Entiende todos los problemas con explicaciones al instante y pasos fáciles de aprender de la mano de expertos reales.